Datapages, Inc.Print this page

  Click to view posters in PDF format.

      Poster 1           Poster 2           Poster 3

 

PSSeismic and Structural Analysis of a Trenton-Black River Hydrothermal Dolomite Reservoir

By

Justine Sagan1 and Bruce Hart2

 

Search and Discovery Article #40129 (2004)

*

1Earth and Planetary Sciences, McGill University, Montreal, Canada; currently Calgary, Alberta.

2Earth and Planetary Sciences, McGill University, Montreal, Canada ([email protected])

 

Abstract

Trenton-Black River reservoirs in the Appalachian Basin are typically associated with fault-related hydrothermal dolomites that are sealed by unaltered host rocks; however, the details of how faulting and fluid flow have interacted remain poorly documented. Integration of 3-D seismic, wireline and production data from Saybrook Field in northeastern Ohio has shown that the productive trend is controlled by a 5-km long, NW-SE oriented basement fault that was probably reactivated during the Taconic Orogeny in Mid- to Late Ordovician. The far-field stresses of this compressional activity caused strike-slip movement of the pre-existing fault to create complex flower structures that branch 1350ft upward into the Trenton-Black River interval. Circular collapse structures within splays of the flower structure are the primary drilling targets. Faults were mapped using amplitude and coherency versions of the seismic data. Curvature analysis of horizons mapped in the seismic data allowed us to constrain further the location and orientation of subtle structures. Fault morphology provides insights into the path of the dolomitizing fluids, whereas the distribution of porosity, and thus the location of the reservoir, has been mapped in 3-D using a seismic attribute study. We integrated wireline log-based measurements of porosity with seismic attributes to predict the distribution of porosity throughout the 3-D volume. Advanced visualization technologies allowed us to integrate faults and porosity predictions, thereby gaining fundamental insights into the relationships between faulting, fluid flow, and reservoir development. Our results and the methodology that we employ have application in analog settings elsewhere.

 

 

uAbstract

  uFigures 1-5

uMethodology

  uFigures 6-14

  uAttributes in porosity prediction

  uFigures 15-17

uConclusions

uReferences

uAcknowledgments

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

uAbstract

  uFigures 1-5

uMethodology

  uFigures 6-14

  uAttributes in porosity prediction

  uFigures 15-17

uConclusions

uReferences

uAcknowledgments

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

uAbstract

  uFigures 1-5

uMethodology

  uFigures 6-14

  uAttributes in porosity prediction

  uFigures 15-17

uConclusions

uReferences

uAcknowledgments

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

uAbstract

  uFigures 1-5

uMethodology

  uFigures 6-14

  uAttributes in porosity prediction

  uFigures 15-17

uConclusions

uReferences

uAcknowledgments

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

uAbstract

  uFigures 1-5

uMethodology

  uFigures 6-14

  uAttributes in porosity prediction

  uFigures 15-17

uConclusions

uReferences

uAcknowledgments

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

uAbstract

  uFigures 1-5

uMethodology

  uFigures 6-14

  uAttributes in porosity prediction

  uFigures 15-17

uConclusions

uReferences

uAcknowledgments

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

Figure Captions (1-5)

Figure 1: Location of the Saybrook 3-D survey in Ashtabula County in the northeast corner of Ohio.

Figure 2: A simplified stratigraphic column for northeastern Ohio from Late Cambrian to Early Silurian (adapted from Larsen, 2000). The Trenton-Black River was deposited primarily as supratidal to subtidal bioclastic carbonates (Middleton et al., 1993).

Figure 3: The synthetic traces and well ties for the two wells with sonic logs, Schoneman and KRCAL. The synthetic correlated well, with correlation coefficients of 0.82 and 0.87, respectively.

Figure 4. a). Sample seismic line with horizon picks shown. Note the small grabens in the basement in the south of the survey and the higher structure to the north. b). Base map for Saybrook, with the cross sections for Figures 7 and 17 shown.

Figure 5. Time structure maps for top of the Trenton (a) and Top of the Basement (b). Both structure maps show a ridge associated with the faulting and productive trend. The Top Basement map shows some NE-SW-trending structures that appear to be small grabens in cross section (see Figure 4). However, these structures are not apparent at the Trenton level.

Return to top.

 

Methodology 

The following steps were taken in the completion of this study: First, the horizons were picked in the well logs; then synthetic seismograms were created to tie the wells to the seismic data. The horizons were then picked in the seismic data, and faults were mapped using a combination of coherency and conventional amplitude seismic displays; the resulting structural features were analyzed. We sought to create a porosity volume for the Trenton-Black River interval using the total average porosity (PHIA), which was generated from the average of the density-porosity and neutron-porosity logs. We used the methodology of Hampson et al. (2001) to identify the best combination of attributes for predicting PHIA. We then trained a neural network to convert the seismic amplitude data to a porosity volume. The porosity volume was combined with the fault mapping in order to examine relationships between porosity and structural features.

 

Figure Captions (6-14) 

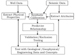Figure 6. A schematic diagram of the workflow followed during this study (adapted from Pearson and Hart, 2004)

Figure 7. a). Stratal slice through the coherency volume 18ms above the Top Basement horizon, with highly coherent reflections shown in white and less coherent in black. The sinuous nature of the basement fault can be seen, along with the step-over faults to the north and south. b). Inline 93 is a transect through one of the positive flower structures that was mapped.

Figure 8. a). A simplified block diagram of helicoidal deformation above a single basement fault (after Mandl, 1988). b). The main Riedel shear shown with the Top Basement structure map; its helicoidal nature is apparent.

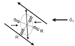Figure 9. The strain ellipse for Saybrook, using the general strike-slip criteria that the primary stress is oriented at 45 degrees to the direction of movement.

Figure 10. Looking down the fault system at Saybrook, at the Trempealeau level and above (Trempealeau time structure is shown). The main synthetic Riedel shear is light green, and the less developed Riedel shears are in darker greens. The en echelon nature of these faults is apparent from this angle, which appears to be consistent with the generalized shear model presented in Figure 13. The inset to the right shows the correct orientation of the entire fault zone in 3-D.

Figure 11. Maximum curvature extracted from the prestack Trenton horizon, draped over the 3D surface. Negative curvature is seen in red and orange, while positive curvature is in blue and green. The main fault ridge is clearly a positive anomaly (convex-up), while well locations (black dots) are located in small negative curvature anomalies (concave-up) along the ridge.

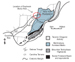Figure 12. Paleogeographic reconstruction from the Mid to Late Ordovician of southeast Laurentia, showing the location of Taconic activity on the northeast edge of the New York Promontory (after Ettensohn et al., 2002).

Figure 13. A generalized left-lateral Riedel shear model (rotated to have the same orientation as Saybrook), in two stages of development: a) initially with only synthetic Riedels (R) and b) later with antithetic (R') (adapted from Mandl, 1988 and Ahlgren, 2001). The regularly spaced extension along sub-seismic antithetic faults combined with minor dip-slip movement may have helped to develop fluid migration pathways.

Figure 14. Graph showing the prediction error and validation error for the multiattribute analysis. While the validation error decreases slightly for predictions using 7 and 8 attributes, 6 is determined to be the optimal number in order to avoid overtraining the data.

 

Attributes in Porosity Prediction 

The attributes used in the porosity prediction were:

RMS amplitude

Perigram

Reflection Strength

Derivative of Instantaneous Amplitude

Integrated Trace

Cosine of Instantaneous Phase

 

Figure Captions (15-17) 

Figure 15. a). The application of the neural network to the training data; the average error was 0.96% and the correlation was 89%. The prediction closely matches the target log (PHIA), except at the bottom of some of the wells where it under-predicted the values. b). Crossplot of the predicted versus the actual values of porosity. The high number of data points is indicative of a volume-based approach, which gives more statistically significant results (Hampson et al., 2001).

Figure 16. a),b). By exporting the created porosity volume into a program that allowed the faults and seismic to be viewed together in 3-D, it was possible better to visualize the relationship between the faults and the predicted porosity. Values below about 5% porosity were made transparent so that the high-porosity (producing) areas could be highlighted. While some noise was predicted by the analysis, c) especially on the edges of the survey, in general the porosity is closely associated with the mapped fault network. The highest porosity values are also concentrated in the areas of intense faulting, especially where the flower structures are located. It was also apparent that although the main fault trend continues at about 122o –302 o, the porosity development did not continue with the trend. Instead the porosity is better developed along the NW left-lateral step-over fault (Figure 7) that trends 105 o -285 o, where there is also a small flower structure.

Figure 17. a). Transects through the porosity volume; high porosity is shown in dark reds, while low porosity is in dark blue and black. The volume was only generated for the interval from the top of the Trenton to the Trempealeau horizon. Inline 93 through the porosity volume with Strong UN #1 shown and Inline 33 with the productive Downes #3 on the left and the non-productive Downes #2 on the right. The porosity development is greatest in the areas between the limbs of the flower structures. b). For comparison, the same inlines are shown in the reflection seismic volume.

 

Conclusions 

The Saybrook fault system is consistent with a left lateral strike-slip model, with the main fault movement accommodated by synthetic Riedel shears. Fluid migration may have been aided by the development of antithetic Riedel shears that formed between the overlapping synthetic Riedel shears (flower structures). This hypothesis is supported by the porosity prediction using seismic attributes that illustrated a clear relationship between high porosity values and areas where there are flower structures in the fault zone. 

Through the combined use of seismic attributes and fault mapping in 3-D, it is apparent that faulting is one of the key controls on dolomitization, and hence porosity development at the Saybrook Field. For plays similar to Saybrook in which the reservoir development is related to a strike-slip fault environment, detailed fault mapping should help to illuminate the impact these structures have had on reservoir development.

 

References 

Ahlgren, S.G., 2001, The nucleation and evolution of Riedel shear zones as deformation bands in porous sandstone: Journal of Structural Geology, v. 23, p. 1203-1214.

Ettensohn, F.R., J.C. Hohman, M.A. Kulp, and N. Rast, 2002, Evidence and implications of possible far-field responses to Taconian Orogeny: Middle-Late Ordovician Lexington Platform and Sebree Trough, east-central United States: Southeastern Geology, v. 41, p. 1- 36.

Hampson, D.P., J.S. Schuelke, and J.A. Quirein, 2001, Use of multi- attribute transforms to predict log properties from seismic data: Geophysics, v. 66, p. 220-236.

Larsen, G.E., 2000 (Hull, D.N., 1990, chief compiler), Generalized column of bedrock units in Ohio: http://www.ohiodnr.com/geosurvey/pdf/stratcol.pdf.

Mandl, G., 1988, Mechanics of tectonic faulting: Models and basic concepts: Elsevier: Amsterdam, Netherlands, 407p.

Middleton, K., M. Coniglio, R. Sherlock, and S. Frape, 1993, Dolomitization of Middle Ordovician carbonate reservoirs, southwestern Ontario: Bulletin of Canadian Petroleum Geology, v. 41, p. 150-163.

Pearson, R.A., and B.S. Hart, 2004 (in press), 3-D seismic attributes help define controls on reservoir development: Case study from the Red River Formation, Williston Basin, in G.P. Eberli, J.L. Masaferro, and J.F. Sarg, eds., Seismic imaging of carbonate reservoirs and systems: American Association of Petroleum Geologists Memoir 81.

 

Acknowledgments 

We thank Pete MacKenzie, formerly with CGAS Inc. for supplying the data used in this project. Funding was provided by an NSERC Discovery Grant to Hart. Software was furnished by Landmark Graphics Corp. and Hampson-Russell Software Services.

 Return to top.